G
remmie’s
F
un
P
ages
edited by Gerald Muus
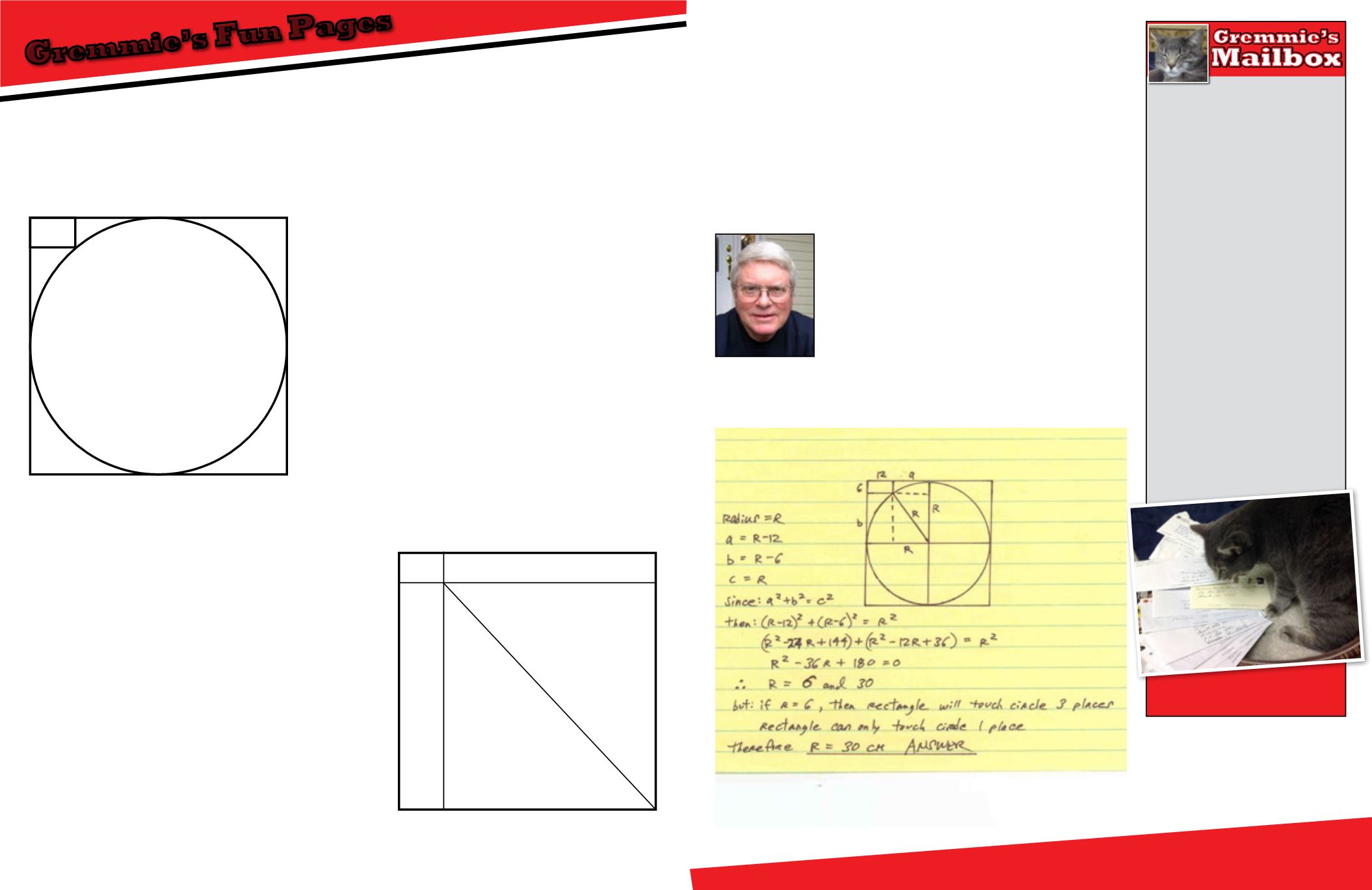
Solution to Last Issue’s Brain Teaser:
We can draw one vertical diameter and one
horizontal diameter in the circle, which divides the
square into four equal smaller squares.
The side of each of the smaller squares is equal to the
radius of the circle.
If r = the radius of the circle, then we can draw a
radius that touches the same point on the circle as the
corner of the rectangle, creating a right triangle as
indicated below.
Using the Pythagorean Theorem, we can calculate the
length of the sides of the right triangle, with “r” as the
hypotenuse:
(r)sq = (r-6)sq + (r-12)sq
(r)sq = (r)sq - 12r + 36 + (r)sq -24r +144
0 = (r)sq - 36(r) + 180
Solution to quadratic equation a(r)sq + b(r) + c is:
r = (-b + sqrt((b)sq-4ac))/2a
a = 1, b = -36, c = 180
r = (36 + sqrt(1296 - 4(1)(180))/2
r = (36 + sqrt(1296 - 720))/2
r = (36 + sqrt(576))/2
r = (36 + 24)/ = 60/2 = 30
As Assistant Editor of Dis-
cover Smith Mountain Lake,
“Gremmie” loves to get mail.
Here she is, busy at work,
looking over the entries in our
puzzle competition.
If you care to drop her a line,
send it to:
Gremlin theWonder Cat
Assistant Editor
Discover Smith Mountain
Lake Magazine
P.O. Box 880
Moneta, VA 24121
Or via e-mail at:
gremmie@discoversmith-
mountainlake.com
Jeff Aldrich of Union Hall submitted the first
correct solution, and he is our winner for the
second issue in a row! Congratulations, Jeff!
Mark Tyson of Moneta also sent in a correct
answer to the puzzle. Mark’s solution diagram
can be seen below.
6
12
r-6
r-12
r-12
Discover Smith Mountain Lake
Summer 2013
22
23


